Home/ Questions/Q 466069
Next
In Process
Wahid Shankar
Asked: 2022-11-08T03:09:16+05:30 In:

In the adjoining figure, seg `XY||` seg `AC`, IF `3AX=2BX` and `XY=9` then find the length of `AC`.
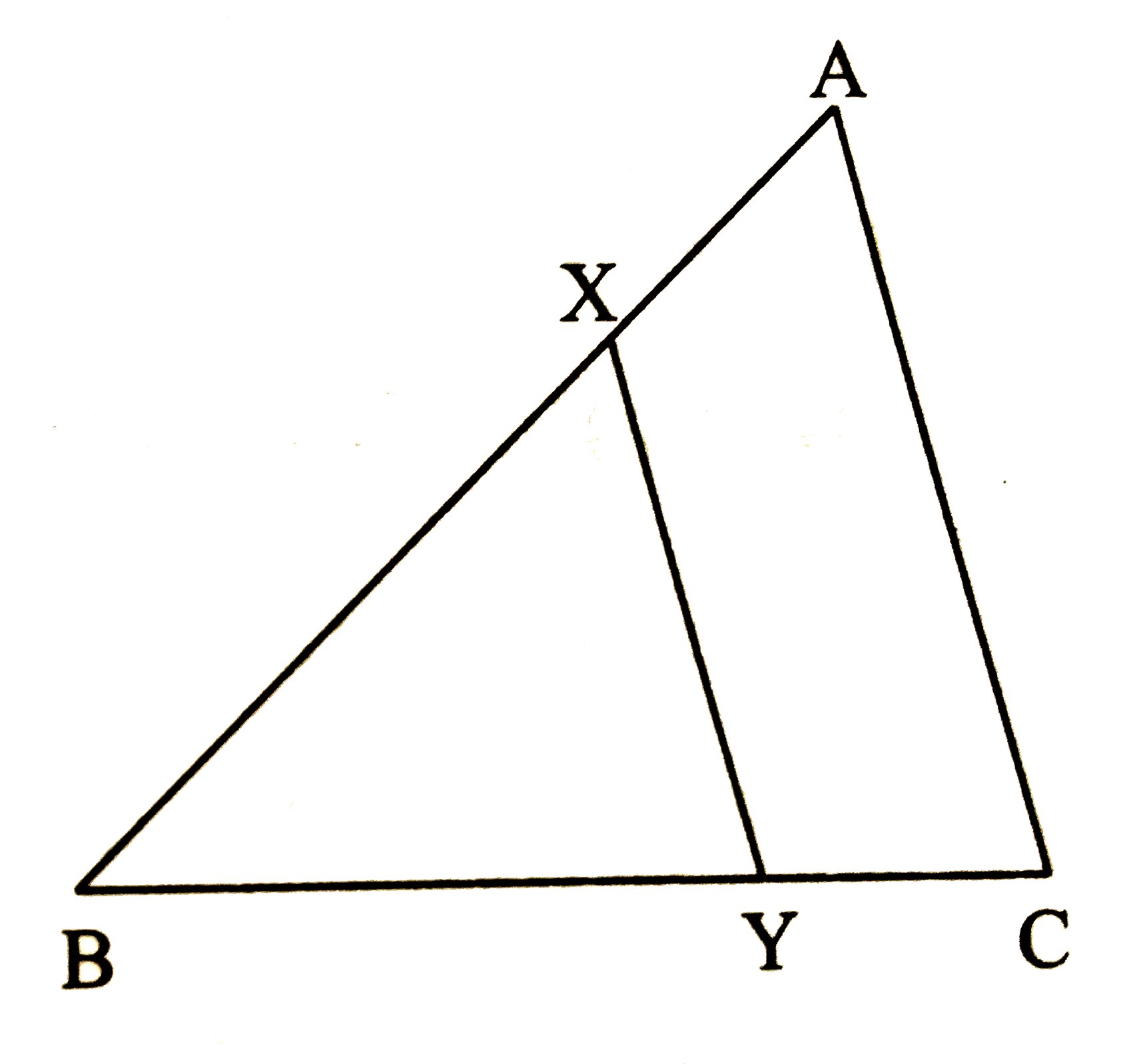
image

In the adjoining figure, seg `XY||` seg `AC`, IF `3AX=2BX` and `XY=9` then find the length of `AC`.
image
Parabola
Leave an answer

Leave an answer

Browse

1 Answer

  1. `3AX=2BX`
    `:.(AX)/(BX)=(2)/(3)`
    By componendo, we get
    `(AX+BX)/(BX)=(2+3)/(3)`
    `(AB)/(BX)=(5)/(3)`
    `:.` by invertendo, we get
    `(BX)/(AB)=(3)/(5)`……`(1)`
    In `DeltaBXY` and `DeltaBAC` ,
    `/_BXY~=/_BAC`…….(Corresponding angles)
    `/_XBY~=/_ABC`…….(Common angle)
    `:.DeltaBXY~DeltaBAC`……(“AA” test of similarity)
    `:.(BX)/(AB)=(XY)/(AC)`……(Corresponding sides of similar triangle)
    `:.(3)/(5)=(9)/(AC)`……..[From `(1)`]
    `:.3xxAC=9xx5`
    `:.AC=(9xx5)/(3)`
    `AC=15`

    • 0